The guessing-box puzzle

Which number is in your box? Using the axiom of choice, we shall find a strategy for the guessing-box puzzle, predicting the contents of various unopened boxes with a shocking degree of reliability

The Grand Guessing-Box Hall

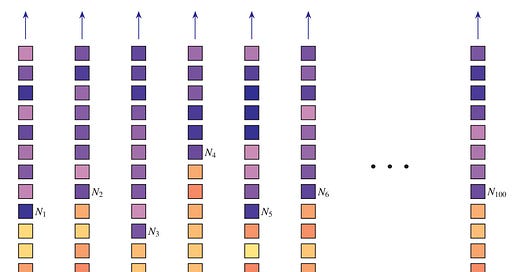
We stand in nervous excitement amongst one hundred fellow mathematicians assembled in the foyer of the Grand Guessing-Box Hall, which houses infinitely many distinctive wooden boxes, each containing a real number. Perhaps one box has π, another √3, and so forth, and perhaps the numbers might appear multiple times or not at all; we can't be sure. The numbers were not necessarily chosen randomly, but might have been chosen so as to thwart an anticipated guessing plan. For in the guessing-box puzzle, we shall indeed aim each to guess the contents of many of the boxes without opening them. A cheer goes up at the announcement that the games shall imminently begin.

According to the established procedure, each mathematician successively will enter the main hall alone, and during the allotted time inspect whichever and as many boxes as desired, opening them up and learning the number inside, and proceeding to further boxes, in a process perhaps determined by the numbers that were previously observed, except that ultimately one box must be left unopened. For this final box, the mathematician will discretely make a prediction of the real number it contains. After the accuracy of the prediction is checked and recorded, the mathematician—whether elated or crushed—will exit to the rear garden, where refreshments will be available, while the boxes are resealed and the room reset to the original state for the next mathematician to enter. We win, as a team, if all our predictions are correct except for at most one of us guessing incorrectly. That is, 99 of us must guess correctly.

Can we do it? We had all seen the room briefly and we have had a few minutes to plan, but naturally no communication will be allowed once the play begins.

Interlude

Enjoy this new installment from The Book of Infinity, a series of vignettes on infinity with all my favorite puzzles and paradoxes, serialized over the past year.

This edition features several varieties of the infinitary guessing-box puzzles, ultimately with uncountably many mathematicians, each making uncountably many guesses about uncountably many boxes, by which we shall achieve shocking solutions via whimsical application of the axiom of choice.

Incredible as it may seem, we can win! There is a strategy ensuring that we shall all predict correctly, except at most one of us guessing in error. The solution will make use of the axiom of choice, much like the infinitary hat puzzle solutions of the previous chapter. And we shall see many further variations of the puzzle, some with uncountably many mathematicians, each making uncountably many predictions about uncountably many unopened boxes.

For the initial puzzle, our winning guessing strategy proceeds like this.